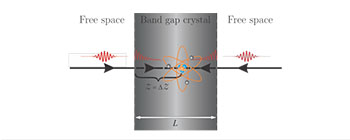
A light source embedded in a photonic band gap crystal (L). The source is located at a depth z = Δz. Credit: MESA+ Institute of Nanotechnology, University of Twente.
When Elahe Yeganegi needed to understand the results of one of her experiments with photonic crystals, she could not find the theory to match her observations. So she worked on developing one.
Yeganegi and three of her colleagues at the MESA+Institute of Nanotechnology of the University of Twente (Netherlands) have calculated how the size of finite photonic crystals controls spontaneous emission (Phys. Rev. B 89, 045123).
Previous theories of photonic-crystal band gaps started with the assumption that the crystals were of infinite size. According to Yeganegi, that premise simplified the analytic calculations greatly. However, the theory also didn't match the results of experiments with actual photonic crystals. Computer simulations of finite-sized crystals went a step further, but did not provide the analytical framework the Twente scientists sought.
Specifically, in an infinite photonic crystal, the absence of vacuum fluctuations forbids the spontaneous emission within the band gap. In other words, its local density of states is zero, independent of the frequency of emission and position within the crystal. However, real crystals have a nonzero local density of states, so the scientists wanted to know how the crystals control emission.
The researchers postulated that in a finite photonic crystal, vacuum fluctuations tunnel into the forbidden region and weaken the band gap. Thus, the local density of states decreases exponentially inside the crystal, depending on frequency, position within the crystal and overall size of the crystal.
Yeganegi believes that the analysis could apply to any finite system, although so far the team has applied the results only to a one-dimensional system.
