Feature
Albert Einstein and the Nature of Light
Einstein’s genius lay in his ability to bridge the gap between radiation in space and radiation-matter interactions. He explained the interaction between light and matter by the absorption and emission of light quanta, thereby explaining several perplexing physical phenomena.
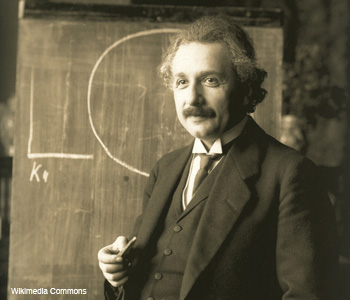Albert Einstein during a lecture in Vienna in 1921.
Albert Einstein was one of the foremost scientists in a century dominated by science. His work influenced our understanding of the physics of the universe, modern electronics and the quantum nature of reality. Perhaps it was his boundless imagination that enabled him to have such a broad reach.
…Log in or become a member to view the full text of this article.
This article may be available for purchase via the search at Optica Publishing Group.
Optica Members get the full text of Optics & Photonics News, plus a variety of other member benefits.